LUNAR OCCULTATIONS & SUPPLEMENT : Part 6During the course of the lunar month the Moon travels through the ecliptic, moving roughly 13.5° each day over the whole 29.3 day synodic period. Naturally, the stars along the ecliptic path will sometimes cross the path of the Moon itself, thus causing the star to be eclipsed or occultated by the approximate 0.5° sized Moon. If the moon followed the ecliptic exactly, we would have repeated monthly occultations of any particular star. Due to the tilt of lunar orbit, star occultations can occur roughly 5.5° either side of the ecliptic. Stars will either disappear or reappear from the lunar limb. For any location on the Earth we can calculate the position of the Moon to high accuracy. As we already know with greater precision the position of the background stars, this also means we can predict the approximate time of an occultation for any place on Earth. Conversely, measuring the exact time of the event determines the Moon’s location. It may come as a surprise, but the lunar position at any time is not known with great precision. Variations are caused by many factors, but the greatest influences of all is the Earth itself. We often think of the Earth as an exact spherical body, but the influence of the continents, the Earth’s shape and rotation cause the Moon to slightly slow or speed up over parts of the Earth. Although these errors might seem small, over time this causes uncertainty in predicting where the moon will be either the future or was in the past. As the Moon has no atmosphere to obscure the starlight, most occultations are instantaneous. They generally merging with the limb, hanging just for a few seconds, then suddenly blinking out. On reemerge, often like a surprise, the star flicks immediately into full brightness. Sometimes poor seeing conditions may interfere in the estimation and decrease the observations accuracy. Over a number of years all lunar occultations are found to occur in cyclic series. Durations between each particular series corresponds to the Saros period of 18 years 11.3 days, when the lunar path will occultate the same bright star. Examples of these cycles include the first magnitude stars are as follows;
Stars lying less than ± 3.93° from the ecliptic have two series every 18.6 years — the regression period of the lunar nodes. The first occultation series visible in the series starts at one of the poles, moves to the aequator, then to the other pole. The next series is the opposite. Between the ecliptic latitudes (β) of ± 3.93° and ± 6.31°, find only one series of occultations each Saros. Usually the time of the first and last occultations in the series of occultations occur at the same pole. Importantly, all occultations can be used to determine future lunar positions. As the Moon travels about one arc minute every two minutes of time, the lunar position can be calculated to an accuracy of about two-hundred metres along the lunar orbit. Achieving this kind of accuracy, the lunar position must be known to about 0.1 arc seconds or better. This is easily measurable by both amateur and professional observations, and have equal weight in value. This requires timing accuracy using either some calibrated stopwatch or chronograph and using small to moderate telescopes, and your eyes to see it. Some observers use the telephone time service and set stopwatches to the beeps while others tune in to short-wave radios to radio time signals transmitted at certain frequencies. A tape recorder is used to record the beeps and the voice and can be played back later. Further discussion on the various time resources and accuracies can be found at the Site; “New Zealand and Australia Time Resources Site”. Sometimes the experienced observer own personal equation must be applied due to differences in the reaction time of the observer that does change between observers and increases in value with the observer’s age. An observer’s personal equation is judged by instrumental techniques or by analysing previous occultations. Commonly this time never exceeds 0.2 to 0.3 seconds of time. Information from the numerous observations made through out many parts of the world are collated together and used to calculate ephemeris time or dynamical time - the well known ΔT. Such observations actually adjust the various time systems used on Earth and gain information on small irregular fluctuations of Earth’s rotation. Sometimes physical details on the stars is also obtained. For example, some stars do not instantly disappear but fade over a second or so. Large red giant stars like Antares fade because of their large true diameters. Others fade because of extended atmospheres, such as Alcyone in the Pleiades. Fades can be attributed to unseen companions in binary systems. Occultation observations are being made all over the world by about one thousand people in some thirty-five nations. Today, and it is not uncommon that national or state astronomical societies co-ordinate observational programs and produce their own predictions based on local circumstances. I.e. The Astronomical Society in New South Wales Inc. (ASNSW), British Astronomical Association (BAA) in England or the Royal Astronomical Association of New Zealand (RASNZ); who all have dedicated sections to this kind of work. P R E D I C T I O N SIn 1623, Bullialdus (1605-1694) was the first person to report seeing a lunar occultation, and this was of the bright star Spica (Alpha Virginis). With the invention of the telescope the possibility of seeing lunar occultations vastly improved, and by about 1700 A.D., lunar occultations began to be observed regularly by astronomers. Most occultations were likely considered a novelty, but this changed when Edmond Halley realised this could have useful merit. He proposed that lunar occultations or the measurable close approach of stars by the Moon could solve the navigation problems plaguing English seafarers in determine the ship’ longitude or positions. There was some debate on whether such observations were feasible on the rocking deck of a ship, and whether it was possible to use astronomical devices to do so. In those days they used quadrant circles, which were large and bulky - and even Halley himself thought this was difficult. In 1731, John Hadley made this more practical in developing a handheld quadrant circle with small optical mirrors and lenses. This was only slightly further developed to become the sextant — the navigation device still used today. Halley was so impressed he declared it sufficiently accurate for the purpose. Lunar position tables were then prepared, so that the idea of occultations became redundant. This occultation method proved also impracticable because of the rarity of these events and predicting them, but in principle was a sound idea. In the beginning of the 19th Century lunar occultations were again in vogue in regards navigation and the positioning of shipping on the oceans. NOTE : Lunar occultation in time would prove to be useful in determining the lunar orbit far more accurately. The advantage was the improved knowledge on the positions of the fixed stars, which held far better accuracy than the lunar position. Today we know there are many causes for the variations in the lunar rate of motion, where occultation can give a very good means of quantifying these changes. The first occultation ephemeris appeared in 1824 in the British Nautical Almanac, whose precise observations were used to enable ships to determine longitudes. These predictions have appeared in various types of ephemeris ever since. At one time these were issued from H.M. Nautical Almanac Office at the Royal Greenwich Observatory, but this service has been discontinued since 1981. Similar predictions continued to be made by the U.S. Naval Observatory, but eventually even these were discontinued in 1992. After 1992 predictions started being made by the now Japanese based, “International Lunar Occultation Centre” (ILOC). The ILOC was originally formed in 1923 in the United States by the I.A.U. (International Astronomical Union), but are now based at the Geodesy and Geophysics Division of the Hydrographic Office in Tokyo, Japan. Monthly predictions were available through an Internet automated computer program ILOC Predictions. but this was discontinued in 2004. Another group also formed in the United States is the “International Occultation Timing Association” (IOTA) at http://www. lunar-occultations.com/iota/iotandx.htm that comprised mainly amateur and professional observers. this body collects, analyses and maintains occultation data - making its data for research. It also makes prediction of future grazes and occultations which is also available on request and will assists observers with their occultation programmes. The need for serious predictions of lunar occultations started in 1937 when a world-wide ephemeris was made available to observers. Here the event times were calculated depending on the size of the telescope and the observer’s latitude and longitude which must be known to about ten metres. Calculations find that most stellar star’s magnitude limits are based on the “Catalogue of 3539 Zodiacal Stars for Equinox 1950.0.” A.P.A.E., 10, Part II (1940), generally known as the Z.C. Catalogue. These stars were given down to 7.5v magnitude and were used as the standard list for the next fifty years or so. Based on these zodiacal stars, the predictions of occultations had certain limitations imposed. The difficulties in seeing the occultations in telescopes were well known, and the resultant predictions were quite severe. Here the limitations followed;
(These last three being dependant on the time of year and the distance the Sun is below the horizon.) For many years these limitations were imposed, and were based on the likely clear visibility of the occultation for professional then amateur observers. During the 1950’s amateur were encourage to do lunar occultations themselves, and these limitation were based on aperture up to around 10cm. In today’s terms, amateurs have access to much larger apertures and more optically efficient telescopes. These limitations do not apply as stringently as they did in the past. Although this is true, the rough estimates of the listings above suggests that the magnitudes maybe lower, but the proportions for the quoted values are the same. Observers could roughly estimate that for ever extra 5cm of aperture you could add about one magnitude fainter than the results above. I.e. A 20cm could have limiting magnitude (or the first four lines above, as 6.5, 5.5, 9.5 and 8.5, respectively. This could be applied to the Full Moon predictions and the daylight/ twilight prediction as well. However, the limits near the horizon could be maintained, as the geography of the location is the greatest hindrance to seeing low occultations. Furthermore, the effects of poor seeing and cloud are much worse during these times. Limitations on these events does not seem to change much with bigger apertures. The occultation tables given below could be abridged to about one-third of the given list. No guarantees are given to see the marginal events, and observers should be aware of the limitations in the listed limitations above. Experience quickly soon finds out the real limitations. In recent years free computer software has become available to be downloaded from the Internet. The best sites to download are either LOW 4.1 from the Dutch Occultation Centre and Occult 4.1.0 produced by Canberra observer David Herald. Both these programs are large in nature but at least have useful various add-ons, which in most instances, are just large addition catalogues and not required by the casual observer. Predictions themselves are very complex. Various parameters must be known each being based on, for example, size of the telescope, the magnitude of the star, the height above sea level, if it occurs on either the bright and dark limb, to the shape of the edge of the moon. Serious amateurs can download these large programs from the Internet, and both can give you listings for your own latitude and longitude. Those new to occultations can use the attached predictions which gives the brighter stars for the year. Both these programs now list many other catalogues list with stars down to 12th or 15th magnitude. A decent sized easily constructed Dobsonian telescope could possible observe many of these. Events are calculated for about ninety standard cities, including, London, Paris, Sydney, Melbourne, Bombay etc. These standard locations are given, so approximate times of events can be made from hundreds of kilometres from the standard station for possible lunar observers. All predictions give two constants, ‘a’ and ‘b’ that can be used to predict the approximate time that the event will occur. Another value ‘c’ is sometimes stated but is rarely used by amateurs.
a : Gives the correction for longitude positions All these corrections are expressed in minutes in position. This method calculates to one-tenth of a minute and is useful to about five-hundred kilometres from the central datum. Errors between locations will do not usually exceed more than two minutes. From the beginning of serious lunar occultations in 1937, roughly 250 000 events have been observed throughout the world. Between 1943 and 1979 there were 42 000 made, by in recent times, the IOLC is receiving approximately 10,000 observations per year. C A L C U L A T I O N STimes for locations away from the standard datum
|
| Deg | 10° | 20° | 30° | 40° | 50° |
| 1° | 0.1 | 0.1 | 0.1 | 0.0 | 0.0 |
| 2° | 0.3 | 0.3 | 0.2 | 0.2 | 0.1 |
| 3° | 0.7 | 0.6 | 0.5 | 0.4 | 0.3 |
| 4° | 1.2 | 1.1 | 0.9 | 0.7 | 0.5 |
| 5° | 1.8 | 1.7 | 1.4 | 1.1 | 0.8 |
| 6° | 3.0 | 2.4 | 2.0 | 1.6 | 1.1 |
| 7° | 4.0 | 3.0 | 3.0 | 2.2 | 1.5 |
| 8° | 5.0 | 4.0 | 4.0 | 3.0 | 2.0 |
| 9° | 6.0 | 5.0 | 5.0 | 4.0 | 2.5 |
Here, roughly estimate the difference in latitude to ± 9° of the station datum, which incidentally covers many of the population centres of east coast Australia. For disappearances ADD the correction value, for reappearances SUBTRACT the correction value to the predicted time. The error here should not exceed one minute along similar longitudes.
This rough correction (Tp ; in minutes.) here is based on equation;
Tp = 0.075 . cos2φ . (Δφ)2
REFERENCE
“Explanatory Supplement to the Astronomical Almanac.” p.293 (1961)
One Standard Station
Times for locations away from the standard datum may be calculated by;
Tp = T1 + ( a × Δλ ) + ( b × Δφ )
Where;
Tp = Predicted Time
T1 =
Calculated time for a Station
Δλ = Difference in Longitude from Station
Δφ = Difference in Latitude from Station
If the location is East, Δλ is Negative, West,
Δλis Positive.
If the location is South, Δφ is Negative, North,
Δφ is Positive.
Example 1
If Sydney location lies at −151°15′
East (-151.254) and −33°54̸ South (-33.9), calculate
the time of occultation of the star φ Piscium on the 18th
September 1989, for the location of the Astronomical Society of New
South Wales Observing Site, Wiruna. Wiruna is located at ;
λ = −149.7670° East, φ = −33.0175°
South.
The time predicted for the standard station is 05h 03.1m AEST and
‘a’
= −1.2,
‘b’
= 1.8.
What is the time of reappearance for Ilford ?
Δλ = −149.767 −
(−151.254) = +1.4870 (As West is +ve)
Δφ = -33.0175 - (−33.9) = +0.8825 (As North is
+ve)
Tp = 05h 03.0m + (−1.2 × 1.487) + (+1.8
× 0.8825)
Tp = 05h 03.0m -1.7 + 1.5
Tp = 05h 02.8m
Hence ;
φ Piscium will be occultated at 05h 02.8m at the observer’s location at Wiruna
Two Standard Stations
If the locations is no more than about 500 kilometres away it is best to use two standard stations, and then calculate the new mid-point values for ‘a’ and ‘b’. It is best to find your location’s latitude and longitude then calculate the value of “q”. As the observer is more likely to do occultation from home, this value may be used for all future calculations — thus simplifying the number crunching.
Once this is known, then you can apply this to the simple new ‘a’ and ‘b’ values. If ‘a’ and ‘b’ is for the nearest station datum, the observer is at ‘a1’ and ‘a1’, with the second datum location lying at ‘a2’, and ‘b2’, then;
q = (φ - φ1) / 2 . (φ2−&phi1)
a = a1 + q . ( a2 − a1 )
b = b1 + q . ( b2 − b1 )
Example 2
If Sydney is -151.2°, −33.9° (‘a1’ and ‘b1’) and Melbourne is −145.0°, −37.8° (a2 and b2), calculate the time of occultation of the star ZC 3211 for the observer’s location −147.5°, −35.2°, if it was predicted to disappear from Sydney at 21h 11.6m ?
Where;
a1 = −1.1, b1 = −2.8,
a2 = −0.7, b2 =
−1.9
φ1 = −33.9° Δλ =
+3.7
φ2 = −37.2° &Deltaφ =
+1.3
What is the time of occultation ?
First, calculate the new ‘a’ and ‘b’ co-officiants for you new location;
q = −35.2-(−33.9) / 2 .
(−37.2−(−33.9)) = −1.3 / 6.6 =
−0.1970
a = −1.1 + −0.1970 × (−0.7−(−1.1))
= −1.179
b = −2.8 + −0.1970 × (−1.9−(−2.8))
= −2.977
Hence;
Tp = T1 + ( a × Δλ
) + ( b × Δλ )
Tp = 21h 11.6m + (−1.179 ×
(−147.5−(−151.2)) + (−2.977 ×
(−33.9 −( −35.2))
Tp = 21h 11.6m + (−1.179 × +3.7) +
(−2.977 × (−33.9 + 35.2))
Tp = 21h 11.6m + −4.36m + −3.87m
Tp = 21h 03.4m
Hence;
ZC 3211 will disappear at 21h 03.4m at the observer’s location of −147.5°, −35.2°
Accuracy Check on Method 2
The results can be checked by so called “linking” which involves a calculation that gives an error estimation. This is calculated from the following equation;
(T1 - T2) - 0.5 . (a1 . Δλ + b1 . Δφ + a2 . Δλ + b2 . Δφ) = ~ 0
Remember that T1 here is the first station and T2 is the second one. This result is expressed to one-tenth of a minute.
Furthermore, calculate the differences between stations by
stations;
Δλ = λ1 − λ2 and; Δφ = φ1 − Δλ2
These values are valid up to about 750 km and those results greater than 0.3 are considered acceptable, though beyond this either recheck the calculations or be observed five minutes before the predicted event to make certain to catch the occultation. For example, the calculation above gives the time of the occultation in Melbourne as 21h 05.0m. I.e. 6.4 minutes. The value in the equation using the given ‘a’ and ‘b’ values for both locations gives -6.48, giving the acceptable value of 0.08 or as rounded to 0.1
Grazing Occultations
These events are both exciting and extremely important. Principally, the observation relies heavily on being place in a certain location on the earth’s surface, where some lunar occultation just scrapes the northern and southern edges of the lunar surface. Limits for events on the Earth are often expressed in an ephemeris as a boundary on a map. When the time approaches (usually one or two days to a month before) more precise calculations are required to narrow the position and the time of the event.
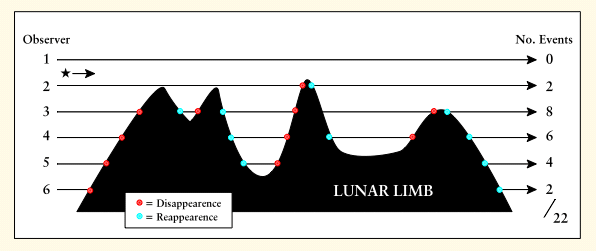
Figure 1 : A Grazing Occultation
Many observers are then positioned along this line near the event’s predicted time. Normally, they are placed within cities at certain street corners or at obvious locations, such as a house, bends in roads or at road junctions. Along the rough lunar limb the star during the graze may disappear and reappear many times as the star skims between the lunar hills and valleys. (Fig. 1) Observers are typically placed over one kilometre or so and roughly perpendicular to the grazing path. Combining the results produces a more accurate lunar profile (See Fig. 2), but more importantly gives the very accurate instantaneous position of the centre of the Moon’s from the multiple events. Calculation of the mean lunar position is very complicated and need to be analysed by some one good with the maths. Accuracy for any grazing occultation when compared to a lunar occultation increases by a factor of ten.
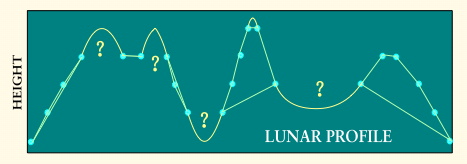
Figure 2 : Lunar Profile From Grazing Occultation
Radio Source Occultations
Sometimes the planets or radio sources will be occultated by the Moon. These planetary events are rare but are very interesting because the whole disk of the planet gradually disappears or reappears from view. Jupiter and Saturn, with their mutual satellites, have events occurring over about ten minutes. Most spectacular is Saturn, as its rings makes it look like some alien spacecraft landing or departing from the lunar surface.
Although useful timings of the planetary limbs can be obtained to determine planetary diameters, much of this has been replaced by interplanetary spacecraft. Asteroidal observations in this instance are also highly important.
Prediction of planetary occultations are given with their stellar counterparts in an ephemeris. Radio sources sometimes are also occultated whose true position of the sources can be accurately determined. Ie. example, the Crab Nebula supernova remnant is the most accurate known radio object.
NOTES
One place which gathers occultation observations is The International Lunar Occultation Centre (ILOC) made by (amateur) astronomers worldwide. You too may send your observations (see Export) to the ILOC or to nominated local organisations. The address of the ILOC is:
International Lunar Occultation Centre
Geodesy and Geophysics Division
Hydrographic Department
Tsukiji 5, Chuo-ku
Tokyo 104 Japan
Telephone: 81-3-35413811
Fax: 81-3-35452885
E-mail: iloc@cue.jhd.go.jp
If you do not have a computer system with installed programs like LOW or OCCULT, you can contact ILOC for predictions. Observations made by you will send you, including if case you did not have one, an ILOC Code a nd the results of the data reduction of your observed data with the so-called O − C results (Observed − Corrected). Then the ILOC sends preliminary reductions of your observations usually within days after they’ve received the data in electronic form. You should preferably send your observations in soon after the first and second half of the year.
Another place worthy of a visit is the Royal Astronomical Society of New Zealand’s Occultation Section (if you have not done so already!). Observers can find the latest information at this website.
Prediction Theories Used
In calculating the occultation predictions, use has been made of the following theories of motion :
ELP2000-85 : Theory of Lunar Motion
(Chapront)
�VSOP87 : Theory of Planetary Motions (for Mercury through
Uranus)
IAU : Theory of Nutation 1985 / Theory of Libration
Disclaimer : The user applying this data for any purpose forgoes any liability against the author. None of the information should be used for either legal or medical purposes. Although the data is accurate as possible some errors might be present. Onus of its use is placed solely with the user.
Last Update : 10th October 2012
Southern Astronomical Delights © (2012)